極坐標 (Polar Coordinate)
國立蘭陽女中數學科陳敏晧老師

在數學領域中，坐標表示法除了直角坐標(Cartesian Coordinate)外，還有極坐標系統，這種系統是將一個點 \(P\) 與中心點 \(O\) (極點pole)連線成 \(\vec{OP}\)，過 \(O\) 點作一向右水平線(極軸pole axis)，以 \(\vec{OX}\) 為始邊，\(\vec{OP}\) 為終邊之有向角 \(\theta\)，

如圖一所示，令 \(r=\overline{OP}\)，若我們用 \((r,\theta)\) 來代表 \(P\) 的位置時，則稱 \((r,\theta)\) 為 \(P\) 之極坐標，其中稱 \(r\) 為「模」(modulus)，而 \(\theta\) 稱為「幅角」(argument)，當 \(0\le \theta<2\pi\) 時，稱為「主幅角」(principal argument)，另外，有向角的定義方式也與廣義角相同，即逆時針旋轉時為正角，順時針旋轉時為負角。 Continue reading →

倍角公式(II) (Double-angle Formulas)
國立蘭陽女中數學科陳敏晧老師

連結：倍角公式(I)

三角函數中的倍角公式，主要有兩類，一類是二倍角公式，一類是三倍角公式，其中二倍角公式主要有：

1. \(\sin 2\theta= 2\sin \theta \cos \theta\)
2. \(\cos 2\theta= {\cos ^2}\theta- {\sin ^2}\theta= 2{\cos ^2}\theta- 1 = 1 – 2{\sin ^2}\theta\)
3. \(\displaystyle\tan 2\theta= \frac{{2\tan \theta }}{{1 – {{\tan }^2}\theta }}\)

這些公式的證明主要是利用正弦與餘弦的和差角公式：

\(\sin \left( {\alpha+ \beta } \right)= \sin \alpha\cos \beta+ \cos \alpha \sin \beta \\\cos \left( {\alpha+ \beta } \right) = \cos \alpha \cos \beta- \sin \alpha \sin \beta\)

若 \(\alpha=\beta=\theta\)，則 \(\sin 2\theta= \sin \theta \cos \theta+ \cos \theta \sin \theta= 2\sin \theta \cos \theta\)，

\(\begin{array}{ll}\cos 2\theta &= \cos \theta \cos \theta- \sin \theta \sin \theta\\&= {\cos ^2}\theta- {\sin ^2}\theta= {\cos ^2}\theta- (1 – {\cos ^2}\theta ) \\&= 2{\cos ^2}\theta- 1 = 2(1- {\sin ^2}\theta ) – 1 = 1 – 2{\sin ^2}\theta\end{array}\)

Continue reading →

半角公式(II) (Half-angle Formulas)
國立蘭陽女中數學科陳敏晧老師

連結： 半角公式(I)

在1580年左右，法國代數學家維塔(François Viète,1540-1603)

曾經提出一個漂亮的正切函數半角公式：$$\displaystyle\frac{{a + b}}{{a – b}} = \frac{{\tan \left( {\frac{{A + B}}{2}} \right)}}{{\tan \left( {\frac{{A – B}}{2}} \right)}}$$。

證明的方法是利用正弦定理及和差化積公式：

$$\begin{array}{ll}\displaystyle\frac{{a + b}}{{a – b}} &=\displaystyle \frac{{2R\sin A + 2R\sin B}}{{2R\sin A – 2R\sin B}} = \frac{{\sin A + \sin B}}{{\sin A – \sin B}} \\&=\displaystyle \frac{{2\sin \left( {\frac{{A + B}}{2}} \right)\cos \left( {\frac{{A – B}}{2}} \right)}}{{2\sin \left( {\frac{{A – B}}{2}} \right)\cos \left( {\frac{{A + B}}{2}} \right)}}=\displaystyle \frac{{\sin \left( {\frac{{A + B}}{2}} \right)/\cos \left( {\frac{{A + B}}{2}} \right)}}{{\sin \left( {\frac{{A – B}}{2}} \right)/\cos \left( {\frac{{A – B}}{2}} \right)}} \\&=\displaystyle \frac{{\tan \left( {\frac{{A + B}}{2}} \right)}}{{\tan \left( {\frac{{A – B}}{2}} \right)}}\end{array}$$

Continue reading →

半角公式(I) (Half-angle Formulas)
國立蘭陽女中數學科陳敏晧老師

三角函數中的半角公式：

\(\displaystyle\sin\frac{\theta}{2}=\pm \sqrt{\frac{{1-\cos\theta }}{2}}\) (\(\pm\) 號依 \(\displaystyle\frac{\theta}{2}\)在第幾象限而定)

\(\displaystyle\cos\frac{\theta}{2}=\pm \sqrt{\frac{{1+\cos\theta }}{2}}\) (\(\pm\) 號依 \(\displaystyle\frac{\theta}{2}\)在第幾象限而定)

\(\displaystyle\tan \frac{\theta }{2}=\pm\sqrt {\frac{{1-\cos \theta }}{{1+\cos \theta }}}= \frac{{\sin \theta }}{{1+ \cos \theta }}= \frac{{1- \cos \theta }}{{\sin\theta }}= \frac{{1+\sin \theta- \cos \theta }}{{1+ \sin \theta+ \cos \theta }}\)

上述半角公式的證明是根據二倍角公式：\(\cos 2\alpha= 2{\cos^2}\alpha- 1= 1- 2{\sin^2}\alpha\)，

令 \(2\alpha=\theta\) 即 \(\displaystyle\alpha=\frac{\theta}{2}\)，移項得 \(\displaystyle 2{\cos ^2}\frac{\theta }{2} = 1+\cos\theta ,2{\sin ^2}\frac{\theta }{2} = 1 -\cos \theta\)，

再移項及開平方得 \(\displaystyle\sin \frac{\theta }{2}=\pm\sqrt{\frac{{1-\cos\theta }}{2}}\)，\(\displaystyle\cos \frac{\theta }{2}=\pm\sqrt{\frac{{1+\cos\theta }}{2}}\)，

將兩式相除得 \(\displaystyle\tan \frac{\theta }{2} = \frac{{\sin \frac{\theta }{2}}}{{\cos \frac{\theta }{2}}}=\frac{{\pm\sqrt {\frac{{1 -\cos \theta }}{2}} }}{{\pm\sqrt {\frac{{1 + \cos \theta }}{2}} }} =\pm\sqrt {\frac{{1 – \cos \theta }}{{1 + \cos \theta }}}\)， Continue reading →

平方關係或畢達哥拉斯三角恆等式 (Pythagorean Trigonometric Identity)
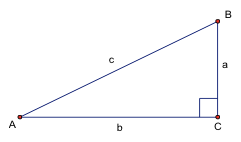
國立蘭陽女中數學科陳敏晧老師

在 \(\Delta ABC\) 中，若 \(\angle ACB=90^\circ\) 且令 \(\theta=\angle BAC\)，如下圖一所示，根據三角函數的定義， 則 \(\sin\theta=\frac{a}{c}\)，\(\cos\theta=\frac{a}{c}\)，\(\tan\theta=\frac{a}{b}\)，\(\cot\theta=\frac{b}{a}\)，\(\sec\theta=\frac{c}{b}\)，\(\csc\theta=\frac{c}{a}\)。

圖一：現行高中數學教科書，銳角三角函數的定義是建立在直角三角形上。

Continue reading →

三角函數值表
國立蘭陽女中數學科陳敏晧老師

三角函數值表：現行的三角函數值表，是將三角函數的近似值算出並製成表格，表從 $$0^\circ$$ 到 $$90^\circ$$ 間(以 $$10’$$ 為單位，$$1^\circ=60’$$)的各種三角函數值，超過 $$90^\circ$$ 或小於 $$0^\circ$$ 的角，再利用廣義角的性質轉換。表中最左一行由上而下呈現的角度是遞增情形，對應最上一列由左而右有 $$\sin$$、$$\cos$$、$$\tan$$、$$\cot$$、$$\sec$$、$$\csc$$ 各個函數符號；表中最右一行由下而上呈現的角度是遞增情形，對應最下一列由左而右印有 $$\cos$$、$$\sin$$、$$\cot$$、$$\tan$$、$$\csc$$ 和 $$\sec$$ 各個函數符號，因此，查表的簡易口訣為「左上右下」，下圖一為三角函數值表的部分表格。 Continue reading →

圓錐曲線的定義作圖
臺北市立西松高中蘇惠玉老師

圓錐曲線的定義

拋物線、橢圓、雙曲線等圓錐截痕有各種不同的定義方式，目前高中教材中選擇的是與焦點與固定長有關的定義方式，分別定義如下：

拋物線：

給定一直線 $$L$$ 及線外一點 $$F$$，若平面上的動點 $$P$$ 滿足到 $$F$$ 點的距離等於到直線 $$L$$ 的距離，即 $$\overline{PF}=d(P,L)$$，則所有動點 $$P$$ 所形成的圖形為拋物線。

橢圓：

給定兩點 $$F_1$$ 與 $$F_2$$，以及一固定值 $$2a$$，其中 $$2a>\overline{F_1F_2}$$，若平面上的動點 $$P$$ 滿足到 $$F_1$$、$$F_2$$ 的距離和等於此固定值，即 $$\overline{PF_1}+\overline{PF_2}=2a$$，則所有動點 $$P$$ 所形成的圖形為橢圓。

雙曲線：

給定兩點 $$F_1$$ 與 $$F_2$$，以及一固定值 $$2a$$，其中 $$2a<\overline{F_1F_2}$$，若平面上的動點 $$P$$ 滿足到 $$F_1$$、$$F_2$$ 的距離差等於此固定值，即 $$|\overline{PF_1}-\overline{PF_2}|=2a$$，則所有動點 $$P$$ 所形成的圖形為雙曲線。

從這樣的定義方式並沒有辦法看出圖形的樣子，在還沒導出標準式之前，也無法藉由描點的方式畫圖。因此，要能夠「接受」這樣的定義方式確實可以畫出所定義的圖形，就必須經由作圖工具的輔助才行。

Continue reading →

動態模擬軌跡方程式
臺北市立西松高中蘇惠玉老師

在高中圓錐曲線的教材中，有一個教學目標為讓學生瞭解何謂軌跡方程式。藉由題目的設計，同時也讓學生理解圓錐曲線的來源，不是只有平面與圓錐的截痕，也不是只有按照定義方式才能得到。但是在學習過程中，學生們並不容易理解何謂動點（或動圓）？何謂軌跡？也很難從題目提供的條件「視覺化」的理解軌跡圖形為何？因此如果教師能夠藉由電腦軟體的模擬效果，將可讓學生輕易地經由「視覺」來理解幾何概念。以下為筆者整理在此單元教學時看過的幾個有關軌跡方程式的問題（問題來源皆為南一版學習講義第四冊），並試著以GeoGebra軟體來作動態模擬。 Continue reading →